One day, Plato asked his teacher Socrates, “What is love? How can I find it?” Socrates answered, “There is a vast wheat field in front. Walk forward without turning back, and pick only one stalk. If you find the most magnificent stalk, then you have found love.” Plato walked forward, and before long, he returned with empty hands, having picked nothing. His teacher asked, “Why did you not pick any stalk?” Plato answered, “Because I could only pick once, and yet I could not turn back. I did find the most magnificent stalk, but did not know if there were any better ones ahead, so I did not pick it. As I walked further, the stalks that I saw were not as good as the earlier one, so! I did not pick any in the end.”
Socrates then said, “And that is LOVE.”
On another day, Plato asked Socrates: “What is marriage? How can I find it?” His teacher answered, “There is a thriving forest in front. Walk forward without turning back, and chop down only one tree. If you find the tallest tree, then you have found marriage”. Plato walked forward, and before long, He returned with a tree. The tree was not bad but it was not tall, either. It was only an ordinary tree, not the best but just a good tree. His teacher asked, “Why did you chop down such an ordinary tree?” Plato answered, “Based on my previous experience, I had walked through the field, but returned with empty hands. This time, I saw this tree, and I felt that it was the first good tree I had seen, so I chopped it down and brought it back. I did not want to miss the chance.”
Socrates then said, “And that is MARRIAGE.
On another day, Plato asked his teacher, “What is life?” Socrates asked him to go to the forest again, allowed back and forth as well, and pluck the most beautiful flower. Plato walked forward. However he hadn’t come back for 3 days. His teacher went to find him. When he saw Plato’s camping in the forest, he asked:” Have you found the most beautiful flower?” Plato pointed a flower near to his camp and answered, “This is the most beautiful flower!” “Why didn’t you take it out?” Socrates asked. “Because if I pick it, it would be drooping. Even though I didn’t pick, it would die in a couple of days for sure. So I had been living by its side while it was blooming. When it’s drooped, I was up to find another one. This is the second most beautiful flower I have found!”
Socrates then said, “You’ve got the truth of LIFE”
“Love” is the most beautiful thing to happen to a person, it’s an opportunity you don’t realize its worth when you have it but only when it’s gone like the field of stalks.
“Marriage” is like the tree you chopped, it’s a compromise; you pick the first best thing you see and learn to live a happy life with it. Having an affair is alluring. It’s like lightning - bright but disappeared so quickly that you cannot catch up with and keep it.
“Life” is to follow and enjoy the every beautiful moment of living. That’s why you should enjoy your life wherever you live.
有一天,古希腊哲学家柏拉图问他的老师苏格拉底什么是爱情,他的老师就叫他先到麦田里,摘一棵全麦田里最大最金黄的的麦穗。期间只能摘一次,并且只可以向前走,不能回头。柏拉图于是照着老师的说话做。结果,他两手空空的走出麦田。老师问他为什么摘不到,他说:“因为只能摘一次,又不能走回头路,其间即使见到一棵又大又金黄的,因为不知前面是否有更好,所以没有摘;走到前面时,又发觉总不及之前见到的好,原来麦田里最大最金黄的麦穗,早就错过了;于是,我什么也没摘到。”
苏格拉底说:“这就是爱情。”
之后又有一天,柏拉图问他的老师什么是婚姻,他的老师就叫他先到树林里,砍下一棵全树林最大最茂盛、最适合放在家作圣诞树的树。其间同样只能摘一次,以及同样只可以向前走,不能回头。柏拉图于是照着老师的说话做。今次,他带了一棵普普通通,不是很茂盛,亦不算太差的树回来。老师问他,怎么带这棵普普通通的树回来。他说:“有了上一次经验,当我走到大半路程,已经感到累了却还两手空空时,我觉得虽然树林里还有很多树,但这棵树还是挺不错的,便砍下来,免得最后又什么也带不出来。”
苏格拉底说:“这就是婚姻。”
又有一天柏拉图又问老师苏格拉底什么是生活,苏格拉底还是叫他到树林走一次。要求是随便走,在途中要取一支最好看的花。柏拉图有了以前的教训又充满信心地出去过了三天三夜,他也没有回来。苏格拉底只好走进树林里去找他,最后发现柏拉图已在树林里安营扎寨。苏格拉底问他:“你找着最好看的花么?” 柏拉图指着边上的一朵花说:“这就是最好看的花。” 苏格拉底问:“为什么不把它带出去呢?” 柏拉图回答老师: “我如果把它摘下来,它马上就枯萎。即使我不摘它,它也迟早会枯。所以我就在它还盛开的时候,住在它边上。等它凋谢的时候,再找下一朵。这已经是我找着的第二朵最好看的花了。”
苏格拉底说: “你已经懂得生活的真谛了。”
爱情给人经历和回忆,之后,婚姻靠的是明智的决定和好好的把握,经过了这些考验,到最后才会明白生活是一种珍惜和守护。
柏拉图麦穗问题的数学解答
现在我们用数学的角度来讨论这个问题。
假设我们碰到的麦穗有 n 个,我们用这样的策略来选麦穗,前 k 个,记住一个最大的麦穗记为 d(可能是重量,也可能是体积),然后 k + 1 个开始,只要大于 d 的,就选择,否则就不选择。
对于某个固定的 k,如果最大的麦穗出现在了第 i 个位置(k < i ≤ n),要想让他有幸正好被选中,就必须得满足前 i - 1 个麦穗中的最好的麦穗在前 k 个麦穗里,这有 k / (i - 1) 的可能。考虑所有可能的 i,我们便得到了前 k 个麦穗作为参考,能选中最大麦穗的总概率 P(k):
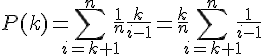
设 k / n = x,并且假设 n 充分大,则上述公式可以改为:

对 x·ln(x) 求导,并令这个导数为 0,可以解出 x 的最优值,它就是欧拉研究的神秘常数的倒数 1 / e.
所以 k = n / e.
如果你想摘取最大的麦穗,假设有 n 个麦穗,你应该先将前 n / e 个麦穗作为参考,然后再 k + 1 个麦穗开始选择比前面 k 个最大的麦穗即可。
e = 2.718281828459
1 / e = 0.36787944117144
其他例子
一、一楼到十楼的每层电梯门口都放着一颗钻石,钻石大小不一。你乘坐电梯从一楼到十楼,每层楼电梯门都会打开一次,只能拿一次钻石,问怎样才能拿到最大的一颗。
首先,这个题目说的,并不能完全拿到最大的钻石。但可以保证拿到最大钻石的概率最大。10 / e = 3.67,向上取整得 4。前四层皆不取,只记下最大的。后面遇到的,只要比前面最大的还大,取之即可。
二、秘书问题。在机率及博弈论上,秘书问题(类似名称有相亲问题、止步问题、见好就收问题、苏丹的嫁妆问题、挑剔的求婚者问题等) 内容是这样的:
要聘请一名秘书,有 n 人来面试。每次面试一人,面试过后便要即时决定聘不聘他,如果当时决定不聘他,他便不会回来。面试时总能清楚了解求职者的适合程度,并能和之前的每个人作比较。问凭什么策略,才使选得到最适合担任秘书的人的机率最大?
References
- 麦穗理论,又名“秘书问题” (1/e 处为最优分割点), http://geek.csdn.net/news/detail/231497